(You may obtain a PDF version of this lab note at Electrodynamic Geodesics) Note: See also Part 3 of this Lab Note, Gravitational and Electrodynamic Potentials, the Electro-Gravitational Lagrangian, and a Possible Approach to Quantum Gravitation, which contains further development.
1. Introduction It has been understood at least since Galileo’s refutation of Aristotle which legend situates at the Leaning Tower of Pisa, that heavier masses and lighter masses similarly-disposed in a gravitational field will accelerate at the same rate and reach the ground after identical times have elapsed. Physicists have come to describe this with the principle that the “gravitational mass” and the “inertial mass” of any material body are “equivalent.” As a material body becomes more massive and so more-susceptible to the pull of a gravitational field (back when gravitation was viewed as action at a distance), so too this increase in massiveness causes the material body in equal measure to resist the gravitational pull. By this equivalence, the result is a “wash,” and so with the neglect of any air resistance, all the bodies accelerate and fall at the same rate. (The other consequence of Galileo’s escapade, is that it strengthened the role of experimental testing, in relation to the “pure thought” upon which Aristotle had relied to make the “obvious” but untested and in fact false argument that heavy objects should fall faster. In this way, it spawned the essence of what we today know as the scientific method which remains a dynamic blend of thought and creativity, with experience and cold, hard numbers derived from measurement of masses, lengths, and times.)
Along his path to developing the General Theory of Relativity (GTR), Albert Einstein made a brief stop in 1911 in an imaginary elevator, to conduct a gedanken in which he concluded that the physical experience of an observer falling freely in a gravitational field before terminally hitting the ground is no different from what was commonly thought of as Newton’s inertial motion in which a body in motion remained in motion unless acted upon by a “force.” (GTR later showed that this was not quite true, the “asterisk” to this insight arising from the so-called tidal forces.) And, he concluded that the force one feels standing on the floor of an elevator in free fall to which a constant force is then applied, is no different from the force one feels when standing on the surface of the earth.
The General Theory of Relativity, in the end, captured inertial motion and its close cousin of free-fall motion in a gravitational field, in the most elegant way, as simple geodesic motion in a curved geometry along geodesic paths which coincide precisely with the paths one observes for bodies moving under gravitational influences. This was a triumph of the highest order, as it placed gravitational theory on the completely-solid footing of Riemannian geometry, and became the “gold standard” against which all other physical theories are invariably measured, even to this day. (“Marble and wood” is another oft-employed analogy.)
However, the question of “absolute acceleration,” that is, of an acceleration which is not simply a geodesic phenomenon of unimpeded free fall through a swathe carved out by geometry, but rather one in which an observer actually “feels” a “force” which can be measured by a “weight scale” in physical contact between the observer and that body which applies the force, is in fact not resolved by GTR. To this day, it is hotly-debated whether or not there is such a thing as “absolute acceleration.” Surely, the forces we feel on our bodies in elevators and cars and standing on the ground are real enough, but the question is whether there is some way to understand these forces — which are impediments to what would otherwise be our own geodesic free fall motion in spacetime under the influence of gravity and nothing more — as geodesic forces in their own right, simply of a different, supplemental, and perhaps more-subtle character than the geodesics of gravitation. That is the central question to be examined in this lab note.
If we think of the “gravitational mass” of a material body more generally as its “interaction mass” for the specific circumstance in which the “interaction” is “gravitational,” then the answer to the question whether the real forces we feel when our bodies are “absolutely” accelerated might still be described in terms of geometric geodesics, may still lurk amidst Galileo’s legendary escapade at Pisa, but with a twist. In this situation, the “interaction mass” of a material body is now inequivalent to its “inertial mass,” because that interaction is now “electrical” rather than “gravitational.” Here, Aristotle has his day, because “electrically-heavier” bodies do fall faster than “electrically-lighter” ones.
How do electrical masses now come into play? When we fail to maintain our gravitational geodesic motion by failing to morph through the floor of the elevator, or when we fail to continue our gravitational free fall by not falling unimpeded through the earth’s surface, it is because we are stopped by the collective electrical repulsion between billions of electrons in our bodies and billions more in the elevator floor or the earth’s ground. It is because the electrical interaction has now trumped the gravitational interaction and taken us off of our gravitational geodesics. Or, perhaps, if we can obtain a geometric insight into electrodynamics, it is because we are now leaving the gravitational geodesic, and the atoms in our body are instead embarking upon a different sort of geodesic path which now coincides with the path that has long been observed as the Lorentz force motion of a charged mass in an electromagnetic field.
In light of the quantum revolution of the 20th century, one other consideration is in order. In this discussion, we are talking not about quantum phenomenon, but about bulk phenomenon which lend themselves to completely classical description. In the same way that a bulk material body follows a geodesic path through gravitation, the question we raise is whether bulk electrical bodies, or large numbers of electrons in an electric field, can also be understood, via their Lorentz force motion, to be following geodesic paths made of “marble” no less fine than the marble with which General Relativity directs the paths of material bodies through spacetime geometry in a manner that coincides precisely with what we observe and measure to be a gravitational path. We want to understand why we don’t fall through the elevator or through the earth. Not only do we want to understand this in a way that avoids contradicting the geodesic principles of gravitation, we want to do so in a way that seamlessly extends these principles in a totally-self consistent way, into the electromagnetic arena.
Five-dimensional theories (or higher), have frequently been a foundation upon which to try to merge classical gravitation with classical electrodynamics. Kaluza and Klein began the trend, Einstein looked favorably on the effort, many others have followed, but to this day, there is as yet no theory which has been fully compelling in all aspects, and which at the same time, is motivated to a fifth dimension in a completely natural way, conservatively based on solid principles of observational physics which are already firmly-established.
2. Using Dirac’s “Gamma-5” to Motivate a Fifth, Timelike Dimension
One of the most important connections in all of physics is given by the Dirac relationship:
, (2.1)
whereby the Dirac matrices,
, are defined so as to reproduce the Minkowski metric tensor
under anticommutation. This relationship not only underlies Dirac’s equation, but also ensures that the Klein-Gordon equation applies to fermions as well as bosons. It is firmly established in all respects, and certainly must be regarded as one of those physical relationships which is made of “marble” over wood.
Also made of “marble,” is the axial Dirac matrix first motivated by Weyl:
, (2.2)
which is defined from matrix-multiplying the other four Dirac matrices, and which has a well-established and rigorously-observed physical meaning in relation to the left- and right-chiral handedness of elementary fermions. We know that when the are sandwiched between Dirac spinors in the form
, the resulting current source density
(also thought of as a probability and flux density) transforms as a four-vector in spacetime. We also know that
is a “pseudo-scalar.” Though there are five such Dirac gamma matrices, only four these are multiplicatively independent.
We will now motivate a five-dimensional spacetime, based on (2.1) and (2.2), in the following way: The five-dimensional spacetime we employ will be one which is defined as a geometry in which and
, taken together, all transform together as a five-vector
, with
. In this five-dimensional space, we employ uppercase Greek indexes. We maintain the lower case
for the usual 4-dimensional spacetime subspace.
The metric tensor for such a five-dimensional geometry, must therefore be formed from the anticommutator of all five of the , similarly to (2.1). That is, if
is to transform as a five-vector, then we must define a five-dimensional, 5×5 Minkowski metric tensor according to:
. (2.3)
Given the well-known anticommutation properties of the five , one can readily deduce that
, and that
. The usual Minkowski metric tensor
is of course preserved in the 16 = 4×4 components of
for which
.. Importantly, because
, we find that this fifth dimension has a timelike, rather than a spacelike signature. Put succinctly: this five-dimensional geometry consists of two timelike and three spacelike dimensions.
We next define infinitesimal coordinate intervals in the usual way, including a fifth interval, that is,
. Because
is known as the “axial” matrix and because it is associated with a timelike metric signature as noted just above, we shall refer to
as the “axial time” coordinate, and will continue to refer to
as the “ordinary time” coordinate. The
coordinates of course retain their role as ordinary space coordinates.
Geometrically, in light of the two timelike dimensions, it will often be very useful to regard time not as a “time line” but as a “time plane.” Thus, following Feynman, we might not only think about worldlines which move forwards and backwards in time, but also which move sideways in time, and at various angles through the time plane. In fact, it is particularly helpful if one draws a vertical coordinate axis for ordinary time orthogonal to a horizontal coordinate axis for axial time
, to represent the “time plane.” Then for material bodies “at rest,”
, one may speak about the “angle” at which their worldlines move through this time plane. As we shall see, this may lead to a solely-geometric way to understand rest mass, electric charge, and electrical Lorentz Force motion, as geodesic motion through curved, non-Euclidean geometry.
The next step is to specify a metric interval for this five-dimensional spacetime. One might regard this as a “flat” spacetime and so define
. However, if our objective is to understand the motions of electrical bodies on the basis of geodesic paths through a geometry, we must take one final step, and allow this five-dimensional geometry to be a curved, non-Euclidean geometry just like that which is used in GTR. Thus, we shall establish a metric tensor
just as in GTR, and specify the weak-field limit according to
, i.e.,
.. We further maintain the usual interval in the 4-dimensional spacetime subspace, using
, and we thereby specify metric intervals in this 5-dimensional spacetime with axial time, according to:
. (2.4)
(As an aside, for completeness, having extended to incorporate curvature and hence gravitation, we should also return to (2.3), and redefine the Dirac Gamma matrices so as to incorporate these curvatures as well. Thus, we now define a new set of Dirac matrices
from the contravariant
, according to:
. (2.5)
These , which are now fields rather than constant matrices, and which approach the usual
in the weak-field limit, now implicitly include gravitational effects. When employed in Dirac’s equation, these
lead to some very interesting ways to interpret the Schwinger magnetic moments as indicative of gravitational effects near the Planck length which give clues as to the true “size” of the elementary fermions, and these may also bear a relationship to the
used in perturbation theory to represent non-divergent perturbative corrections. But these are topics for an entirely different paper. Let’s return to the main thread of discussion by returning to (2.4).)
3. A Possible Geometric Interpretation of Rest Mass
In applying (2.4), we extend all of the customary GTR relationships from four to five dimensions. Thus, is a symmetric tensor, inverses are specified by
(thus the
and
are used to lower and raise indexes), the covariant derivative of the metric tensor is defined by
, the 5-D Christoffel connections are
, hence
, and the covariant derivative of a first rank vector
is
.
Now, let’s use algebraic manipulation to rewrite (2.4) in both of the following forms:
and (3.1)
. (3.2)
Using a velocity four-vector , these can be combined to obtain:
. (3.3)
If we define a momentum four-vector for a mass m in the usual way, and contrast (3.3) to the equation
for the energy-momentum of an on-shell mass
, we see that
in (3.3) plays a role identical to that of the mass
in
. In the mass shell equation, m is of course introduced “by hand,” because the most one can deduce from the four-dimensional metric equation
is
; then we need to multiply through by a mass m which we simply take out of “thin air” based on our empirical knowledge that masses exist in nature. In contrast, in (3.3), the
multiplier of
arises totally out of the five-dimensional geometry, with nothing introduced “by hand.”
is marble, and m is wood.
We can capture this very-telling correspondence, by writing:
. (3.4)
That is, in some way to be further determined, the rest mass of a material body appears to be proportional to the ratio of to
, and so may have a simple geometric foundation based on the trajectory of a worldline in the
time plane. Now, we are ready to examine the geodesics of this five-dimensional geometry.
4. The Geodesic Equation in Five Dimensions
The five-dimensional calculation to follow is analogous to one way of deriving the geodesic equation in four dimensions, though we will in any event carry out the calculation in full a) to help the reader review the basic role of the metric equation as the first integral of the equation of motion b) to convince the reader that the 5-D geodesic equation is in fact correct, and c) to establish a careful discipline about working in five dimensions, making sure that all of our calculations are carried out in a 5-covariant rather than only a 4-covariant manner.
We return to the metric equation (2.4), and now rewrite this, again via trivial algebraic rearrangement, as:
. (4.1)
Taking the covariant derivative of each side, employing , renaming indexes, commuting, and using
, enables us to then write:
. (4.2)
The covariant derivative , which we substitute into (4.2). After some rearranging of terms, and assuming that
, we can write:
. (4.3)
Finally, we contract the and
indexes, do some index renaming, and obtain the geodesic equation in 5-Dimensions:
. (4.4)
This looks just like the four-dimensional equation, but for the indexes summing over all five dimensions rather than four, and the rather than
in the denominators. But, we can multiply through fully by
, and rewrite (4.4) as:
. (4.5)
Now, let us work with (4.5) above.
Equation (4.5) is a set of five independent equations. Let’s separate it into the four spacetime equations represented by:
. (4.6)
and the single axial-dimension equation:
. (4.7)
Equation (4.6) is for , which is the observed acceleration of a worldline in the observed four dimensions of spacetime. But, the five-dimensional summation in
in (4.6) adds some new terms to the usual gravitational geodesic equation. Specifically, (4.6) expands to:
(4.8)
which on account of , we consolidate with some index renaming to:
(4.9)
5. Geodesic Motion of a Charged Mass in an Electromagnetic Field
Now, it is time to contrast (4.9) above to the Lorentz Force Law when taken together with the gravitational geodesic equation. This is, for example, set forth in equation (20.41) of Gravitation by Misner, Wheeler and Thorne:
. (5.1)
At the outset, let us set aside the term in (4.9). We can do, this, for example, by setting
. We also move the
in front of the
, so that (4.9) now becomes:
(5.2)
Now, we contrast (5.1) directly with (5.2).
The term in (5.2) is the “marble” of geometry, while the corresponding term
is the “wood” of an empirically-derived relationship. However, comparing these terms, we can give a totally geometric footing to the Lorentz force law if we note the proportionalities:
, and (5.3)
. (5.4)
Combining (3.4), , with (5.3), additionally yields:
(5.5)
In fact, if we substitute the proportionalities in (5.3) and (5.4) into (5.1) as if they were equalities, then the geodesic equation (5.2), totally-based in geometry, is the Lorentz force law. Lorentz force motion now appears to be motion along a geodesic, just like gravitational motion. Additionally, this geodesic motion appears to introduce an absolute acceleration, and hence “force,” because of the inequality of electrical and inertial mass, which via (5.3) has its origins in the geometric statement . This geometric statement, is now at the root of the “force,” and hence “absolute acceleration,” experienced by a charged mass in an electromagnetic field. Yet, this force and absolute acceleration, still is a form of geodesic motion described by (5.2), and so rests fully upon the “marble” of geodesic motion through curved geometry.
In fact, let’s take this geometric understanding of inertial mass and electric charge even a step further. Consider a material body viewed at rest, by setting , hence
. Then, according to (2.4):
. (5.6)
Further, take the weak field approximation where ,
. Now, (5.6) becomes:
. (5.7)
Draw and
as orthogonal axes, where
is vertical and
is horizontal (sideways axial time). From (5.7),
is clearly the hypotenuse. Define an angle
. Now, in this weak field
rest frame
, we can write (3.4), (5.3), and (5.5), respectively, as:
. (5.8)
(5.9)
(5.10)
In this way, gravitational and inertial mass, as well as electric mass (charge), obtain a totally geometric interpretation in terms of the angle of a worldline through the time plane. Referring especially to (5.9), movement through ordinary time contributes to gravitational and inertial mass, movement through axial time
contributes to electrical mass, and real “force” and “absolute acceleration” arises from angular movement through the time plane in which a worldline projects both
and
components.
6. Symmetric Gravitation and Antisymmetric Electrodynamics: Can they be Compatible?
Now, let us turn back to the association in (5.4). One of the fundamental difficulties which has been encountered by physicists attempting to unify classical electrodynamics with GTR, is that the former is an antisymmetric field theory while the latter is symmetric. How to combine “oil” and “water” in this way has perplexed physicists for over a century. So, the question arises, does the relationship
provide a path for a seamless and internally-consistent union of these two theories?
As it stands, is a mixed tensor, and it would be better to raise this into contravariant form where we can clearly examine the consequences of having an antisymmetric field strength tensor
. However, now that we are in 5-D, we have to be careful that we are raising indexes properly, using the full
and not just its four-dimensional subset. To do this, we need to recognize that in 5-D, (5.4) should be generalized to
, which means that there are four additional, independent components in
(assuming we maintain an antisymmetric field strength by requiring that
). We are not at this juncture concerned about what these new components might be; the only reason for using
rather than
is to make sure we handle the raising of the lower index properly in 5-dimensions.
So, going into 5-D, and using , we rewrite (5.4) as:
. (6.1)
Although unconcerned for now about the extra components in , we shall follow the customary path and regard
as a totally-antisymmetric tensor, thereby extending this basic property of electrodynamics to these extra components, whatever they may be. That is, we continue to employ the condition
.
Combining with (6.1) now lets us write:
. (6.2)
This lets us express the antisymmetric field strength relation completely in terms of certain relationships involving first derivatives of the gravitational potential, as expressed via the metric tensor. Now, let us reduce this.
From (6.2), we can rename indexes and use the symmetry of the metric tensor to write:
, (6.3)
which further reduces with some index changes and the symmetry of the metric tensor to:
. (6.4)
This is an alternative way of saying that .
We can further simplify this using the inverse relationship , which we can differentiate with respect to the 5th dimension to obtain
, i.e.,
. This can then be used to reduce (6.4) to the very simple:
. (6.5)
The above, , is a purely geometric statement completely equivalent to
. The symmetric field theory of gravitation is fully compatible with the antisymmetric field theory of electrodynamics, so long as we require that
.
7. Do Maxwell’s Equations Become Components of Einstein’s Gravitational Field Equation?
We have shown the possibility that Lorentz force motion might be described as simple geodesic motion in a five-dimensional spacetime with axial time, and that the inequality of electrical and inertial mass which causes one to “feel” a force and prevents one from falling through the floor of Einstein’s elevator or into the earth’s core, may well emanate from the simple proportionality . But equations of motion are only one part of a complete field theory. The other part is a specification of how the “sources” of that theory influence the “fields” originating from those sources. In a complete theory, the equations of motion then describe motion through the fields originating from the sources.
To complete the field theory which we have motivated thus far, one therefore would also need to also examine the Einstein equation in five dimensions:
, (7.1)
as well as the Riemann Identity:
, (7.2)
to see if among their new axial (index = 5) components, one might find the Maxwell equations , and
.
In five dimensions, one would of course specify the Riemann tensor in the usual way, albeit with an extra “5” index. That is:
. (7.3)
Now, let’s consider the component of this equation, that is:
. (7.4)
The second term in the above, with explicit substitution of is given by:
. (7.5)
This is equal to zero, as a consequence of , equation (6.5), which is the same thing as
, and because ordinary derivatives commute. Thus, by virtue of
a.k.a.
, (7.4) simplifies to:
, (7.6)
consisting of only three terms.
Now, we make use of generalized from (5.4), to rewrite (7.6) as a proportionality, in terms of the field strength tensor, as such:
. (7.7)
What is absolutely fascinating, and of enormous eventual import, is that this expression for the mixed field strength tensor is identical to its gravitationally-covariant derivative
. From here, we can get to both of Maxwell’s equations almost immediately.
First, let’s contract (7.7) down to the Ricci tensor, and use the proportionality liberally to eliminate the minus sign, as such:
, (7.8)
where is the five-dimensional, covariant electric source current. Because
, this can be raised into mixed form with some index renaming as:
. (7.9)
Note that , and so
, because
by virtue of
, that is,
.
Now, we can return to Einstein’s equation (7.1), for and
, and use (7.9) as well as
, to write:
, (7.10)
This is the first of Maxwell’s equations, for the field of an electric charge. We find, in particular, that is a four-vector situated along the axial components of the energy momentum tensor. The electric current, which is an electrical source density, is now also simply part and parcel of the generalized gravitational source
!
What about Maxwell’s magnetic equation? Here, we lower all indexes in (7.7) and then use the symmetry to rewrite (7.7) as:
. (7.11)
Then, we turn to some more geometric “marble,” namely the Riemann identity (7.2). Taking the component of this identity, we write:
, (7.12)
We may then consider the spacetime subset equations, to write:
, (7.13)
Now, Maxwell’s magnetic equation also rests on geometric “marble.”
Maxwell’s electrodynamics in this manner, becomes fully unified with Einstein’s gravitation. The equation for an electric source, , is specified in geometry as:
. (7.14)
The magnetic equation is specified in geometry as:
, (7.15)
Finally, the geodesic equation , which includes the Lorentz force law, is specified in geometry as equation (4.9):
. (7.16)
(We note, as an aside, that a.k.a.
implies that
. Other than to match the Lorentz force law term for term, see (5.1) and (5.2), there is no apparent fundamental reason why we must have
. If
, the final term in (7.16) is an extra term in the Lorentz force law, and using
from (5.9), this term is of the form
, and so includes the coupling ratio
. If
, then this term drops out entirely, and we revert to the exact comparison made between (5.1) and (5.2). Additionally, if
, and given that
so
, this means taken together that
, so that
= constant throughout the five-dimensional spacetime geometry. If we presume that the five-geometry is locally, asymptotically flat and therefore can always be transformed into “geodesic coordinates” at a single 5-dimensional event, then because
in geodesic coordinates (i.e., at a single “event,”) it must also be +1 everywhere else. So the condition
would require that
, everywhere.)
Irrespective of the ultimate disposition of , all of the foregoing does appear to place Maxwell’s electrodynamics onto the solid geometric footing of Einstein’s gravitational theory. Even the inequivalence of electrical and inertial mass, and the real, measurable forces and the absolute accelerations which accompany this, are nevertheless the result of material bodies pursuing geodesic worldlines through a five-dimensional spacetime geometry.
Hi Jay,
by tag surfing i stumbled over your blog and i will most likely return. Very interesting ideas and you are certainly able to present them arrestingly.
I had not the time to read the whole entry, but you use the 5th gamma matrix to get a 5-dimensional representation of the clifford algebra. We use the same approach in the Randall-Sundrum Mmodel. The problem we encounter is that because of this in odd dimensional spacetime there is no projection operator. So we are not able to define chiral fermions. We deal with this by orbifolding the extra dimension. I don’t want to explain this further. Just to draw your attention on the problem… you probably already thought about.
keep up the good work.
-martin
Comment by martinbauer — February 6, 2008 @ 11:19 am |
Hi Martin:
I presume the chiral projection operators you refer to are 1 +/- gamma^5? But I am not sure why these would be thought to disappear in an odd-dimensional spacetime.
Would you please send me or point out, something that pinpoints the root of the problem you have in mind, and I’ll see if perhaps some solution seems apparent.
Thanks,
Jay.
email to: jyablon@nycap.rr.com
Comment by Jay R. Yablon — February 6, 2008 @ 6:57 pm |
Hi Jay,
You will not be able to build these projection operators in odd spacetimes. Generally is defined as the product of all “lower” gammas. This gives a projection operator for even dimensional spacetime and 1 for odd dimensions (it’s
is defined as the product of all “lower” gammas. This gives a projection operator for even dimensional spacetime and 1 for odd dimensions (it’s 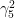 in 5 dimensions).
in 5 dimensions).
So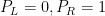 or vice versa. You could use the “old”
or vice versa. You could use the “old”  to build it, but then again you need
to build it, but then again you need ![\left[\gamma _{5} ,\gamma _{\mu } \right]=0](https://s0.wp.com/latex.php?latex=%5Cleft%5B%5Cgamma+_%7B5%7D+%2C%5Cgamma+_%7B%5Cmu+%7D+%5Cright%5D%3D0&bg=ffffff&fg=000000&s=0&c=20201002) to get kinetic terms in the lagrangian which couple left handed fermions to left handed , but you already have
to get kinetic terms in the lagrangian which couple left handed fermions to left handed , but you already have  .
.
In your case, its even worse. Cause …
…
You can look it up at sundrums lecture : http://arxiv.org/abs/hep-th/0508134
theres is some supersymmetry book also, but i cant remember which one right now. will tell you later.
martin
Comment by martinbauer — February 7, 2008 @ 6:07 am |
To do the dirty deed in an odd spacetime you can add an extra degree of freedom to the Clifford algebra that is not a part of the algebra. Replace the four gamma matrices with the symbols x, y, z, t to allow me to avoid tex. Add a fifth gamma matrix s.
Sure enough, the product xyzst is an object that squares to +1 and commutes with everything in the algebra. It’s natural to assume that it is therefore equal to 1 but you don’t have to do that. This is entirely up to the user. Personally, I think you should leave it as a non unit and that makes (1 +- xyzst)/2 perfectly good as a projection operator.
An alternative construction, and one that I worked with for quite some time, is to begin with an even Clifford algebra, like the Dirac algebra with x, y, z, and t, and add a single extra degree of freedom to it. The extra degree of freedom is assumed to commute with everything else in the algebra and square to 1. That will give you an algebra that is isomorphic to that generated by {x,y,z,s,t} and it explicitly defines the (1+xyzst)/2 projection operator as non trivial.
Eventually an expert on the geometric algebra versions of Clifford algebra pointed out to me that what I was doing was isomorphic to the usual C(4,1) so I quit doing that and just used C(4,1) instead. They make perfectly good projection operators.
It is also easy to give a physical justification for adding an extra degree of freedom that commutes with everything else in the algebra. Suppose you wish to define a theory that has non commutative degrees of freedom for the spatial components but is Newtonian in that time is not included in with the spatial degrees of freedom. The natural number of dimensions to take is 3. For the spatial degrees of freedom you get the Pauli algebra.
The Dirac algebra arose from looking for a square root for the Klein-Gordon equation. The massless Klein-Gordon (which is appropriate for theories that split the particles into massless chiral halves) arises naturally in perfectly Newtonian circumstances without spacetime. For example, earth quake waves are governed by it. In this case, the time coordinate is not a part of the geometry, so when you take a square root of it, there’s no (relativistic) motivation to make the time coordinate act like the others. However, the massless Klein-Gordon equation (and any other wave equation) needs two degrees of freedom in time. So if you do not treat time like space you will instead have to split it into two equations that are distinct and coupled. They look something like this:
[tex]D \psi = \phi, D\phi = \psi[/tex]
where D is your Dirac operator without a gamma_0. So this means that instead of using just psi or phi, what you end up with is a vector that contains \psi and \phi as separate components. To melt them into the same object, just define a commuting vector “T” which satisfies TT = 1, and write [tex]\Psi = \psi + T\phi[/tex]. Then you can take the above two coupled linear equations and combine them into a single linear equation:
[tex]D \Psi = 0[/tex]
In doing this, you can use the projection operators (1 +- T)/2 to split the \Psi back into its components. It’s perfectly physical, correct mathematically, and there is nothing wrong with it, rock on.
Comment by carlbrannen — February 11, 2008 @ 12:58 pm |
[…] Note: This Lab Note picks up where Lab Note 2, Part 2, left off, following section 7 thereof. Equation numbers here, reference this earlier Lab […]
Pingback by Lab Note 2, Part 3: Gravitational and Electrodynamic Potentials, the Electro-Gravitational Lagrangian, and a Possible Approach to Quantum Gravitation « Lab Notes for a Scientific Revolution (Physics) — February 14, 2008 @ 1:39 am |
Hi Martin:
As regards your comment #3, I was curious if you felt that Carl provided a satisfactory answer in #4? (I will try to decipher and fix your “does not parse” equations.)
Just saw your new post over at http://martinbauer.wordpress.com/. Your analogy to pirates looking for treasure and not having a great map is a good one.
Carl, by the way, has is own blog over at http://carlbrannen.wordpress.com/.
Best regards,
Jay,
Comment by Jay R. Yablon — February 14, 2008 @ 11:00 am |
Hi Martin:
I fixed up your non-printing latex equations in comment #3. I understand all you said, but don’t see a problem. Maybe I am missing something, but here is how I see it:
I am using a 5-D metric tensor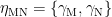 . (Though I work with curved
. (Though I work with curved  , the Minkowski metric tensor will suffice for this discussion.)
, the Minkowski metric tensor will suffice for this discussion.)
You say “Generally is defined as the product of all “lower” gammas.” Do I take this to mean that in 5-D, I have to define yet another
is defined as the product of all “lower” gammas.” Do I take this to mean that in 5-D, I have to define yet another  and use this for projections?
and use this for projections?
You say “This gives a projection operator for even dimensional spacetime and 1 for odd dimensions (it’s in 5 dimensions).” Exactly how do you calculate that the projection operator is
in 5 dimensions).” Exactly how do you calculate that the projection operator is  in 5 dimensions?
in 5 dimensions?
I looked at Sundrum’s lecture at http://arxiv.org/abs/hep-th/0508134 . Thanks for the link. In his (3.1), he defines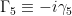 Presumably, this is to force a spacelike signature upon the fifth dimension, i.e., + – – – -, which seems to be the penchant in 5-D theories. I just go with the flow and use the “old”
Presumably, this is to force a spacelike signature upon the fifth dimension, i.e., + – – – -, which seems to be the penchant in 5-D theories. I just go with the flow and use the “old”  , which is what accounts for my + – – – + signature, i.e., for a timelike fifth dimension. As my paper shows, there are advantages that arise from a second timelike dimension, including the fact that mass and charge can be characterized by virtue of their “angle” of travel through time and we can specify the Lorentz force law as geodesic motion, etc. Does that difference perhaps account for the problem you are envisioning? It looks like it does, because, e.g.,
, which is what accounts for my + – – – + signature, i.e., for a timelike fifth dimension. As my paper shows, there are advantages that arise from a second timelike dimension, including the fact that mass and charge can be characterized by virtue of their “angle” of travel through time and we can specify the Lorentz force law as geodesic motion, etc. Does that difference perhaps account for the problem you are envisioning? It looks like it does, because, e.g.,  and
and  are very different projection terms than
are very different projection terms than  and
and  , and the latter get real funny when you take complex conjugates.
, and the latter get real funny when you take complex conjugates.
You say “You could use the “old” to build it,” and in fact, I do simply use the old
to build it,” and in fact, I do simply use the old  as the projection in the usual way. Why not? That is:
as the projection in the usual way. Why not? That is:  as always. I define
as always. I define  and
and  , as always. Thus, when we project from a Fermion wavefunction
, as always. Thus, when we project from a Fermion wavefunction  , we have
, we have 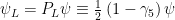 and
and  . Is there a reason why I am compelled not to make these choices?
. Is there a reason why I am compelled not to make these choices?
The adjoint is , and, of course,
, and, of course,  . So,
. So,  and
and  . Then, the Fermion couplings work as follows:
. Then, the Fermion couplings work as follows:
When there is no sandwiched,
sandwiched,  and
and 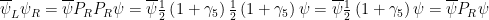 which uses
which uses  and
and  . Further,
. Further,  and
and  , which uses
, which uses  .
.
When there is a sandwiched, then
sandwiched, then 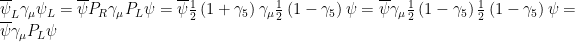 and
and  . Further,
. Further,  and
and  .
.
All of this seems as it should be, to me. Can you please point out where I might be missing something?
Thanks,
Jay.
Comment by Jay R. Yablon — February 14, 2008 @ 12:23 pm |
Hi Carl and Jay,
First, there s a mistake in my first comment. You can delete the 5th sentence.
The question is: are there chiral representations of the Clifford algebra in odd spacetime dimensions (p+q=n odd, no matter how many spaces, times). And the answer is simple : There are none.
you can look it up in a lot of books (i.e. Cornwell has an exhaustive discussion in the appendix ).
You need another, fifth , 4×4 matrix, anticommuting with each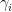 you already have. It is easy to check that the only possibility you have is to use some multiple of
you already have. It is easy to check that the only possibility you have is to use some multiple of 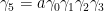 , a some c-number. So
, a some c-number. So 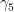 is part of your dirac algebra already.
is part of your dirac algebra already.
Now you try to define projections. To define projection operators you need a 4×4 matrix anticommuting with all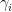 (also
(also 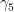 now). There is no such matrix left (= no additional degree of freedom thats not part of the algebra) . That is, because the Clifford algebra provides a basis for the space of 4×4 real matrices. If there’d be another it would be a linear-combination, plus the demand to anticommute with all the others –> it needs to be a product of all
now). There is no such matrix left (= no additional degree of freedom thats not part of the algebra) . That is, because the Clifford algebra provides a basis for the space of 4×4 real matrices. If there’d be another it would be a linear-combination, plus the demand to anticommute with all the others –> it needs to be a product of all 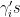 , therefore
, therefore 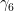 .
.
you can’t use the old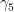 . It commutes with
. It commutes with 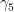 (jay, all your calculations fail if you put
(jay, all your calculations fail if you put 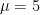 ).
). as you said above will yield
as you said above will yield  (another way: it will commute with every element of the algebra and is therefore 1 by Schurs lemma).
(another way: it will commute with every element of the algebra and is therefore 1 by Schurs lemma).
Try to define a
Carl, the expert on geometric algebra must be joking with you, or he is far from beeing an expert. C(4,1) as every other clifford algebra (group) over an odd spacetime does not allow for a projective operator.
To have chiral fermions you need even spacetime dimension or orbifolding the extradimension.
Best,
martin
Comment by martinbauer — February 15, 2008 @ 1:16 pm |
Hi Martin, Thanks for your comment #8. The Cornwell link is in error — it points to one of my own posts. Can you please provide a corrected link. Thanks. Jay.
PS: Update: Martin sent me a corrected link to Cornwell, now fixed in #8. Jay.
Comment by Jay R. Yablon — February 15, 2008 @ 4:54 pm |